Writing And Balancing Chemical Equations Worksheet
5.1: Writing and Balancing Chemical Equations
-
- Last updated
- Save as PDF
- Page ID
- 98771
Skills to Develop
- Derive chemical equations from narrative descriptions of chemical reactions.
- Write and balance chemical equations
Video \(\PageIndex{1}\): An introduction to writing chemical equations.
The preceding unit introduced the use of element symbols to represent individual atoms. When atoms gain or lose electrons to yield ions, or combine with other atoms to form molecules, their symbols are modified or combined to generate chemical formulas that appropriately represent these species. Extending this symbolism to represent both the identities and the relative quantities of substances undergoing a chemical (or physical) change involves writing and balancing a chemical equation. Consider as an example the reaction between one methane molecule (CH4) and two diatomic oxygen molecules (O2) to produce one carbon dioxide molecule (CO2) and two water molecules (H2O). The chemical equation representing this process is provided in the upper half of Figure \(\PageIndex{1}\), with space-filling molecular models shown in the lower half of the figure.
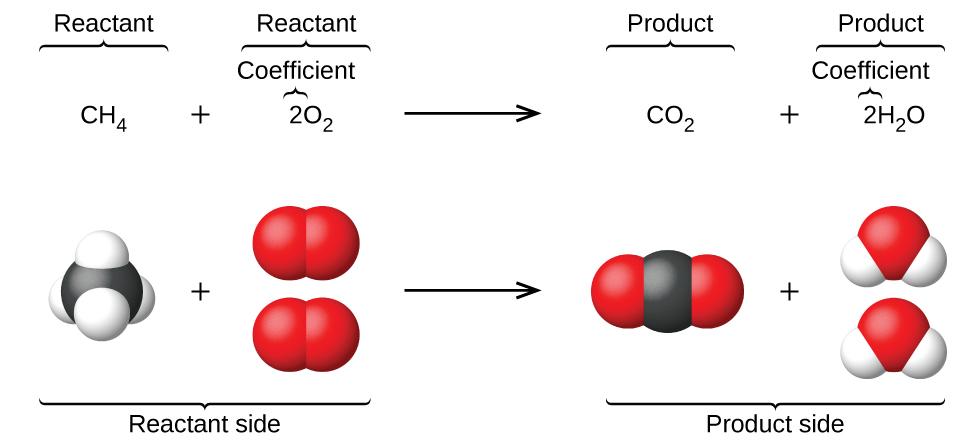
Figure \(\PageIndex{1}\): The reaction between methane and oxygen to yield carbon dioxide and water (shown at bottom) may be represented by a chemical equation using formulas (top).
This example illustrates the fundamental aspects of any chemical equation:
- The substances undergoing reaction are called reactants, and their formulas are placed on the left side of the equation.
- The substances generated by the reaction are called products, and their formulas are placed on the right sight of the equation.
- Plus signs (+) separate individual reactant and product formulas, and an arrow
(⟶) separates the reactant and product (left and right) sides of the equation. - The relative numbers of reactant and product species are represented by coefficients (numbers placed immediately to the left of each formula). A coefficient of 1 is typically omitted.
It is common practice to use the smallest possible whole-number coefficients in a chemical equation, as is done in this example. Realize, however, that these coefficients represent the relative numbers of reactants and products, and, therefore, they may be correctly interpreted as ratios. Methane and oxygen react to yield carbon dioxide and water in a 1:2:1:2 ratio. This ratio is satisfied if the numbers of these molecules are, respectively, 1-2-1-2, or 2-4-2-4, or 3-6-3-6, and so on (Figure \(\PageIndex{2}\)). Likewise, these coefficients may be interpreted with regard to any amount (number) unit, and so this equation may be correctly read in many ways, including:
- One methane molecule and two oxygen molecules react to yield one carbon dioxide molecule and two water molecules.
- One dozen methane molecules and two dozen oxygen molecules react to yield one dozen carbon dioxide molecules and two dozen water molecules.
- One mole of methane molecules and 2 moles of oxygen molecules react to yield 1 mole of carbon dioxide molecules and 2 moles of water molecules.
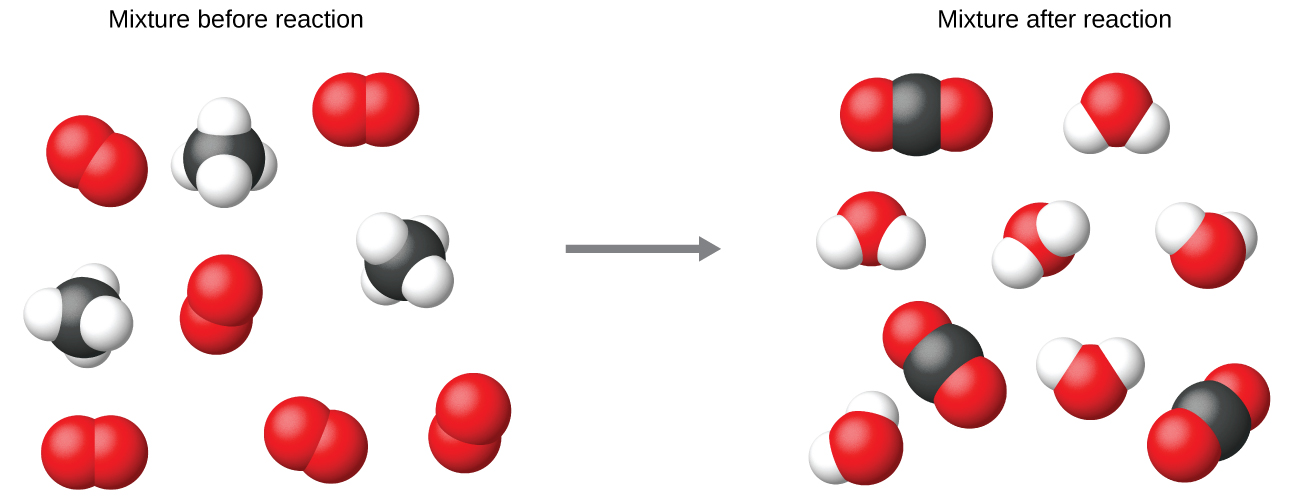
Figure \(\PageIndex{2}\): Regardless of the absolute number of molecules involved, the ratios between numbers of molecules of each species that react (the reactants) and molecules of each species that form (the products) are the same and are given in the chemical equation.
Balancing Equations
Video \(\PageIndex{2}\): An introduction to counting atoms in a chemical equation.
When a chemical equation is balanced it means that equal numbers of atoms for each element involved in the reaction are represented on the reactant and product sides. This is a requirement the equation must satisfy to be consistent with the law of conservation of matter. It may be confirmed by simply summing the numbers of atoms on either side of the arrow and comparing these sums to ensure they are equal. Note that the number of atoms for a given element is calculated by multiplying the coefficient of any formula containing that element by the element's subscript in the formula. If an element appears in more than one formula on a given side of the equation, the number of atoms represented in each must be computed and then added together. For example, both product species in the example reaction, \(\ce{CO2}\) and \(\ce{H2O}\), contain the element oxygen, and so the number of oxygen atoms on the product side of the equation is
\[\left(1\: \cancel{\ce{CO_2} \: \text{molecule}} \times \dfrac{2\: \ce{O} \: \text{atoms}}{ \cancel{\ce{CO_2} \: \text{molecule}}}\right) + \left( \cancel{ \ce{2H_2O} \: \text{molecule} }\times \dfrac{1\: \ce{O}\: \text{atom}}{\cancel{ \ce{H_2O} \: \text{molecule}}}\right)=4\: \ce{O} \: \text{atoms}\]
The equation for the reaction between methane and oxygen to yield carbon dioxide and water is confirmed to be balanced per this approach, as shown here:
\[\ce{CH4 +2O2\rightarrow CO2 +2H2O}\]
| Element | Reactants | Products | Balanced? |
|---|---|---|---|
| C | 1 | 1 | 1 = 1, yes |
| H | 4 | 2 | 4 = 4, yes |
| O | 2 | (1 | 4 = 4, yes |
A balanced chemical equation often may be derived from a qualitative description of some chemical reaction by a fairly simple approach known as balancing by inspection. Consider as an example the decomposition of water to yield molecular hydrogen and oxygen. This process is represented qualitatively by an unbalanced chemical equation:
\[\ce{H2O \rightarrow H2 + O2} \tag{unbalanced}\]
Comparing the number of H and O atoms on either side of this equation confirms its imbalance:
| Element | Reactants | Products | Balanced? |
|---|---|---|---|
| H | 1 | 1 | 2 = 2, yes |
| O | 1 | 1 | 1 ≠ 2, no |
The numbers of H atoms on the reactant and product sides of the equation are equal, but the numbers of O atoms are not. To achieve balance, the coefficients of the equation may be changed as needed. Keep in mind, of course, that the formula subscripts define, in part, the identity of the substance, and so these cannot be changed without altering the qualitative meaning of the equation. For example, changing the reactant formula from H2O to H2O2 would yield balance in the number of atoms, but doing so also changes the reactant's identity (it's now hydrogen peroxide and not water). The O atom balance may be achieved by changing the coefficient for H2O to 2.
\[\ce{2H2O \rightarrow H2 + O2} \tag{unbalanced}\]
| Element | Reactants | Products | Balanced? |
|---|---|---|---|
| H | 2 | 1 | 4 ≠ 2, no |
| O | 2 | 1 | 2 = 2, yes |
The H atom balance was upset by this change, but it is easily reestablished by changing the coefficient for the H2 product to 2.
\[\ce{2H_2O \rightarrow 2H2 + O2} \tag{balanced}\]
| Element | Reactants | Products | Balanced? |
|---|---|---|---|
| H | 2 | 2 | 4 = 4, yes |
| O | 2 | 1 | 2 = 2, yes |
These coefficients yield equal numbers of both H and O atoms on the reactant and product sides, and the balanced equation is, therefore:
\[\ce{2H_2O \rightarrow 2H_2 + O_2}\]
Video \(\PageIndex{3}\): A review of balancing chemical equations, with extra practice problems.
Example \(\PageIndex{1}\): Balancing Chemical Equations
Write a balanced equation for the reaction of molecular nitrogen (N2) and oxygen (O2) to form dinitrogen pentoxide.
Solution
First, write the unbalanced equation.
\[\ce{N_2 + O_2 \rightarrow N_2O_5} \tag{unbalanced}\]
Next, count the number of each type of atom present in the unbalanced equation.
| Element | Reactants | Products | Balanced? |
|---|---|---|---|
| N | 1 | 1 | 2 = 2, yes |
| O | 1 | 1 | 2 ≠ 5, no |
Though nitrogen is balanced, changes in coefficients are needed to balance the number of oxygen atoms. To balance the number of oxygen atoms, a reasonable first attempt would be to change the coefficients for the O2 and N2O5 to integers that will yield 10 O atoms (the least common multiple for the O atom subscripts in these two formulas).
\[\ce{N_2 + 5 O2 \rightarrow 2 N2O5} \tag{unbalanced}\]
| Element | Reactants | Products | Balanced? |
|---|---|---|---|
| N | 1 | 2 | 2 ≠ 4, no |
| O | 5 | 2 | 10 = 10, yes |
The N atom balance has been upset by this change; it is restored by changing the coefficient for the reactant N2 to 2.
\[\ce{2N_2 + 5O_2\rightarrow 2N_2O_5}\]
| Element | Reactants | Products | Balanced? |
|---|---|---|---|
| N | 2 | 2 | 4 = 4, yes |
| O | 5 | 2 | 10 = 10, yes |
The numbers of N and O atoms on either side of the equation are now equal, and so the equation is balanced.
Exercise \(\PageIndex{1}\)
Write a balanced equation for the decomposition of ammonium nitrate to form molecular nitrogen, molecular oxygen, and water. (Hint: Balance oxygen last, since it is present in more than one molecule on the right side of the equation.)
- Answer
-
\[\ce{2NH4NO3 \rightarrow 2N2 + O2 + 4H2O} \nonumber\]
It is sometimes convenient to use fractions instead of integers as intermediate coefficients in the process of balancing a chemical equation. When balance is achieved, all the equation's coefficients may then be multiplied by a whole number to convert the fractional coefficients to integers without upsetting the atom balance. For example, consider the reaction of ethane (C2H6) with oxygen to yield H2O and CO2, represented by the unbalanced equation:
\[\ce{C_2H_6 + O_2 \rightarrow H_2O + CO_2} \tag{unbalanced}\]
Following the usual inspection approach, one might first balance C and H atoms by changing the coefficients for the two product species, as shown:
\[\ce{C_2H_6 + O_2 \rightarrow 3H_2O + 2CO_2} \tag{unbalanced}\]
This results in seven O atoms on the product side of the equation, an odd number—no integer coefficient can be used with the O2 reactant to yield an odd number, so a fractional coefficient,
\[\ce{C2H6 + 7/2 O2\rightarrow 3H2O + 2CO2} \nonumber \]
A conventional balanced equation with integer-only coefficients is derived by multiplying each coefficient by 2:
\[\ce{2C2H6 +7O2\rightarrow 6H2O + 4CO2}\]
Finally with regard to balanced equations, recall that convention dictates use of the smallest whole-number coefficients. Although the equation for the reaction between molecular nitrogen and molecular hydrogen to produce ammonia is, indeed, balanced,
\[\ce{3N2 +9H2\rightarrow 6NH3}\]
the coefficients are not the smallest possible integers representing the relative numbers of reactant and product molecules. Dividing each coefficient by the greatest common factor, 3, gives the preferred equation:
\[\ce{N2 + 3H2\rightarrow 2NH3}\]
Phet Simulation
Use this interactive tutorial for additional practice balancing equations.
State Symbols in Chemical Equations
Video \(\PageIndex{4}\): How to use state symbol to give more information in your chemical equations.
The physical states of reactants and products in chemical equations very often are indicated with a parenthetical abbreviation following the formulas. Common abbreviations include s for solids, l for liquids, g for gases, and aq for substances dissolved in water (aqueous solutions, as introduced in the preceding chapter). These notations are illustrated in the example equation here:
\[\ce{2Na (s) + 2H2O (l) \rightarrow 2NaOH (aq) + H2(g)} \]
This equation represents the reaction that takes place when sodium metal is placed in water. The solid sodium reacts with liquid water to produce molecular hydrogen gas and the ionic compound sodium hydroxide (a solid in pure form, but readily dissolved in water).
Special conditions necessary for a reaction are sometimes designated by writing a word or symbol above or below the equation's arrow. For example, a reaction carried out by heating may be indicated by the uppercase Greek letter delta (Δ) over the arrow.
\[\ce{CaCO3}(s)\xrightarrow{\:\Delta\:} \ce{CaO}(s)+\ce{CO2}(g)\]
Other examples of these special conditions will be encountered in more depth in later units.
Key Concepts and Summary
Video \(\PageIndex{5}\): An overview of balancing chemical equations in the context of stoichiometry.
Chemical equations are symbolic representations of chemical and physical changes. Formulas for the substances undergoing the change (reactants) and substances generated by the change (products) are separated by an arrow and preceded by integer coefficients indicating their relative numbers. Balanced equations are those whose coefficients result in equal numbers of atoms for each element in the reactants and products. Chemical reactions in aqueous solution that involve ionic reactants or products may be represented more realistically by complete ionic equations and, more succinctly, by net ionic equations.
Glossary
- balanced equation
- chemical equation with equal numbers of atoms for each element in the reactant and product
- chemical equation
- symbolic representation of a chemical reaction
- coefficient
- number placed in front of symbols or formulas in a chemical equation to indicate their relative amount
- molecular equation
- chemical equation in which all reactants and products are represented as neutral substances
- product
- substance formed by a chemical or physical change; shown on the right side of the arrow in a chemical equation
- reactant
- substance undergoing a chemical or physical change; shown on the left side of the arrow in a chemical equation
Contributors
-
Paul Flowers (University of North Carolina - Pembroke), Klaus Theopold (University of Delaware) and Richard Langley (Stephen F. Austin State University) with contributing authors.Textbook content produced by OpenStax College is licensed under a Creative Commons Attribution License 4.0 license. Download for free at http://cnx.org/contents/85abf193-2bd...a7ac8df6@9.110).
- Adelaide Clark, Oregon Instititute of Technology
- Fuse School, Open Educational Resource free of charge, under a Creative Commons License: Attribution-NonCommercial CC BY-NC (View License Deed: https://creativecommons.org/licenses/by-nc/4.0/)
- Crash Course Chemistry, References and image licenses for this episode can be found in the Google document here: http://dft.ba/-51j; Crash Course is a division of Complexly and videos are free to stream for educational purposes.
Feedback
Have feedback to give about this text? Click here.
Found a typo and want extra credit? Click here.
Writing And Balancing Chemical Equations Worksheet
Source: https://chem.libretexts.org/Courses/Oregon_Institute_of_Technology/OIT%3A_CHE_201_-_General_Chemistry_I_(Anthony_and_Clark)/Unit_5%3A_Transformations_of_Matter/5.1%3A_Writing_and_Balancing_Chemical_Equations
Posted by: alvaresbeelty.blogspot.com

0 Response to "Writing And Balancing Chemical Equations Worksheet"
Post a Comment